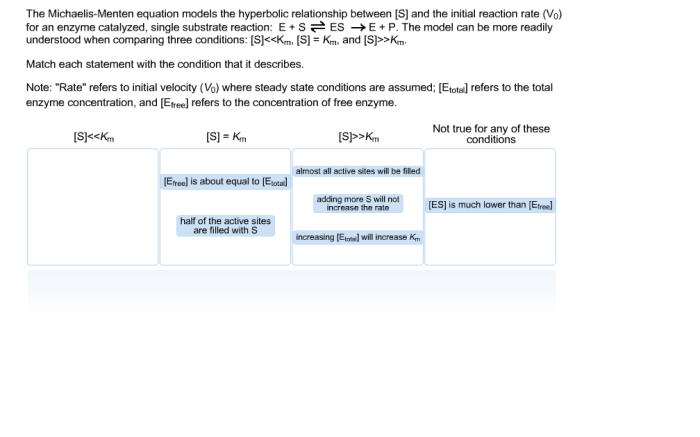
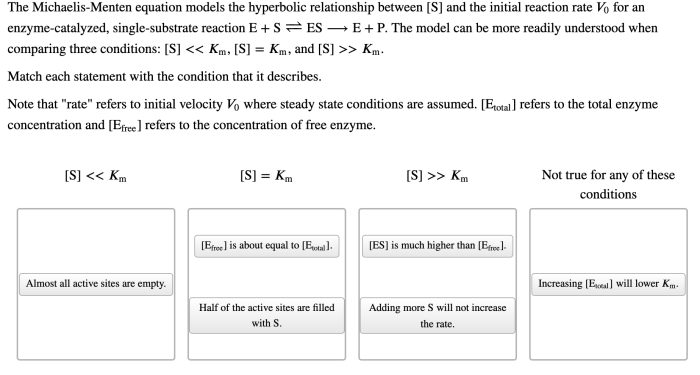
The Michaelis-Menten equation models the hyperbolic relationship between enzyme velocity and substrate concentration, providing a cornerstone for understanding enzyme kinetics. This equation plays a pivotal role in unraveling the intricate mechanisms of enzymatic reactions, paving the way for advancements in fields ranging from biochemistry to pharmacology.
The mathematical elegance of the Michaelis-Menten equation stems from its ability to capture the saturation behavior of enzymes, a phenomenon observed when the enzyme’s active sites become fully occupied by substrate molecules. This saturation effect gives rise to the characteristic hyperbolic curve that defines the Michaelis-Menten relationship, offering valuable insights into enzyme kinetics and its applications.
The Michaelis-Menten Equation
The Michaelis-Menten equation is a mathematical model that describes the relationship between the velocity of an enzyme-catalyzed reaction and the concentration of the substrate. It is one of the most important equations in enzyme kinetics and is used to determine the kinetic parameters of enzymes, such as Vmax and Km.
Mathematical Form of the Michaelis-Menten Equation
The Michaelis-Menten equation is given by the following equation:
v = (Vmax
[S]) / (Km + [S])
where:
- v is the velocity of the reaction
- Vmax is the maximum velocity of the reaction
- Km is the Michaelis constant
- [S] is the concentration of the substrate
Example of Using the Michaelis-Menten Equation
The Michaelis-Menten equation can be used to calculate the kinetic parameters of an enzyme by fitting the equation to experimental data. The following is an example of how the Michaelis-Menten equation can be used to calculate the Vmax and Km of an enzyme:
- Measure the velocity of the reaction at a series of different substrate concentrations.
- Plot the velocity data as a function of the substrate concentration.
- Fit the Michaelis-Menten equation to the data using a nonlinear regression analysis.
- The Vmax and Km values can be obtained from the fitted parameters.
The Hyperbolic Relationship
The Michaelis-Menten equation models a hyperbolic relationship between the velocity of an enzyme-catalyzed reaction and the concentration of the substrate. This relationship is due to the fact that the enzyme becomes saturated with substrate at high substrate concentrations.
Graphical Representation of the Hyperbolic Relationship
The following graph shows a typical hyperbolic relationship between the velocity of an enzyme-catalyzed reaction and the concentration of the substrate:
[Image of a hyperbolic graph]
The graph shows that the velocity of the reaction increases rapidly at low substrate concentrations, but then levels off at high substrate concentrations. This is because the enzyme becomes saturated with substrate at high substrate concentrations, and there are no more free enzyme molecules to catalyze the reaction.
Applications of the Michaelis-Menten Equation

The Michaelis-Menten equation is used in a variety of applications in enzyme kinetics, including:
- Determining the kinetic parameters of enzymes
- Designing enzyme inhibitors
- Understanding the regulation of enzyme activity
Determining the Kinetic Parameters of Enzymes
The Michaelis-Menten equation can be used to determine the kinetic parameters of enzymes, such as Vmax and Km. These parameters can be used to characterize the enzyme’s activity and to understand how it is regulated.
Designing Enzyme Inhibitors, The michaelis-menten equation models the hyperbolic relationship
The Michaelis-Menten equation can be used to design enzyme inhibitors. Enzyme inhibitors are compounds that bind to enzymes and reduce their activity. They can be used to treat a variety of diseases, such as cancer and HIV/AIDS.
Limitations of the Michaelis-Menten Equation
The Michaelis-Menten equation is a simplified model of enzyme kinetics. It does not account for a number of factors that can affect the velocity of an enzyme-catalyzed reaction, such as:
- Substrate inhibition
- Allosteric regulation
- Cooperative effects
Substrate Inhibition
Substrate inhibition occurs when the enzyme becomes inhibited by high concentrations of the substrate. This can occur when the substrate binds to the enzyme’s active site and blocks the binding of other substrate molecules.
Allosteric Regulation
Allosteric regulation occurs when the enzyme’s activity is regulated by the binding of a molecule to a site on the enzyme that is distinct from the active site. Allosteric regulators can either activate or inhibit the enzyme’s activity.
Cooperative Effects
Cooperative effects occur when the binding of one substrate molecule to the enzyme’s active site affects the binding of other substrate molecules. Cooperative effects can either positive or negative.
General Inquiries: The Michaelis-menten Equation Models The Hyperbolic Relationship
What is the significance of the Michaelis-Menten equation in enzyme kinetics?
The Michaelis-Menten equation provides a mathematical framework for understanding the relationship between enzyme velocity and substrate concentration, allowing researchers to determine kinetic parameters such as Vmax and Km.
How does the Michaelis-Menten equation model the hyperbolic relationship between enzyme velocity and substrate concentration?
The Michaelis-Menten equation captures the saturation behavior of enzymes, where the enzyme velocity increases with increasing substrate concentration until it reaches a maximum velocity (Vmax) when all enzyme active sites are saturated.
What are the limitations of the Michaelis-Menten equation?
The Michaelis-Menten equation assumes a simple, one-substrate reaction and does not account for factors such as substrate inhibition, allosteric regulation, or cooperative effects.